Saturday, 20 July 2013
Saturday, 13 July 2013
Men's Tennis Logic Puzzle Walkthrough
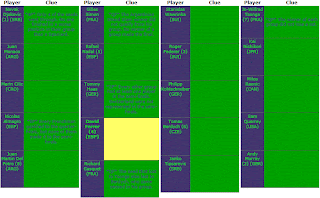
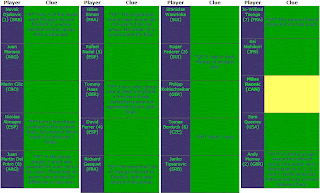
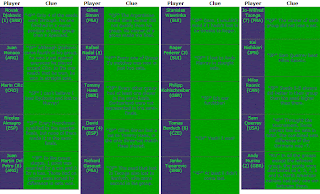
1) Djokovic says that no-one finished in a worse position in
their group than a Spaniard. There are three Spaniards in the grid, Almagro,
Nadal and Ferrer. Nadal and Ferrer are in the same group, so we cannot work out
Nadal and Ferrer’s positions, but we do know that Almago must have finished in
fifth place in his group.
2) Every Frenchman reached the quarter-finals, and progressed
no further. Therefore, Simon, Gasquet were the two qualifiers in Group B. As we
know from Djokovic’s clue that no-one finished lower in their group than a
Spaniard, Tommy Haas has to have come third in his group.
3) Juan Martin Del Potro is the Argentinian Grand Slam
winner, who must have come third in his group, as that is the lowest non-qualification
spot left in Group A.
4) There are two seeds in Group D, Tsonga and Murray. We know
that Murray must have lost to Tsonga. As Tsonga qualified, and no winner of a
group lost a set in the group stages, Murray must have come second, as he later
played, and lost to Berdych. Putting Berdych and Murray into the tournament
tree, we can work out where they met. Murray was in Quarter-Final 3, and
Berdych in Quarter-Final 4. The winners of Quarter-Finals 3&4 could not
meet until the final, so as Murray lost to Berdych, Murray must have been the
runner-up, and Berdych the champion.
5) Only four of the seeds qualified to the knockout stages.
As none of the seeds from Groups A or B qualified for it, all of the seeds from
Groups C and D made the knockout stages. Switzerland was not represented in the
semi-finals, so Roger Federer must have been knocked out in the quarter-finals.
6) There are two North American players, Raonic and Querrey.
One win was shared between them, so as the points in Group D were distributed
4, 3, 2, 1 and 0, Raonic and Querrey must have finished 4th and 5th
in some order, one having defeated the other. Therefore, Nishikori must have
finished third in his group.
7) As Sam Querrey beat Milos Raonic, so Querrey came 4th
and Raonic 5th in their group.
8) There are two semi-final spots left, both of which must be
occupied by the two Group A qualifiers, as there were none from Group B and one
each from Group C and D.
9) Raonic tells us that seeds 5-8 always did better in their
group than seeds 1-4. This means that Ferrer must have come last in his group
and Nadal fourth.
10) Every one of Group 3’s matches was won in two sets. This
means that going by the fact that the points were distributed 4, 3, 2, 1 and 0,
each placing in the group had the following Sets Win/Loss score – 1st:
8/0; 2nd: 6/2; 3rd: 4/4; 4th: 2/6 and 5th:
0/8. Swiss players lost six sets in total. Roger Federer must have won his
group, as Berdych came second, so he won every set and lost only 2, when he
lost to Murray. To make six sets lost in total by Swiss players, Wawrinka must
have come third, so he lost 4 sets.
11) The sets difference for the Germans was -7. Tommy Haas
must have won two and lost two, with one of the losses having to be Simon, who
came second in the group. Haas therefore won two matches 2-0, lost one match
1-2 and lost the other match 0-2, meaning his set difference was 5-4. To make
the combined sets difference for the Germans -7, Kohlschreiber must have had a
sets difference of -8. The only way he could have achieved this was to come
last in the group, so Kohlschreiber was fifth placed.
12) Tipsarevic must have taken the final place, fourth.
13) Done! Congratulations!
Subscribe to:
Posts (Atom)